Gothine 03/04
research iterations by Daniel Koehler, made with processing & punktier, gpu integration by Christoph Zimmel.


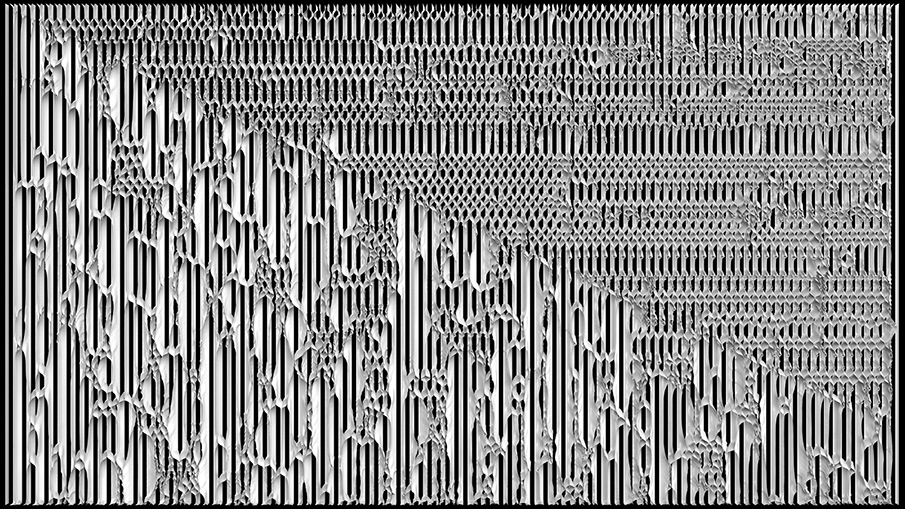
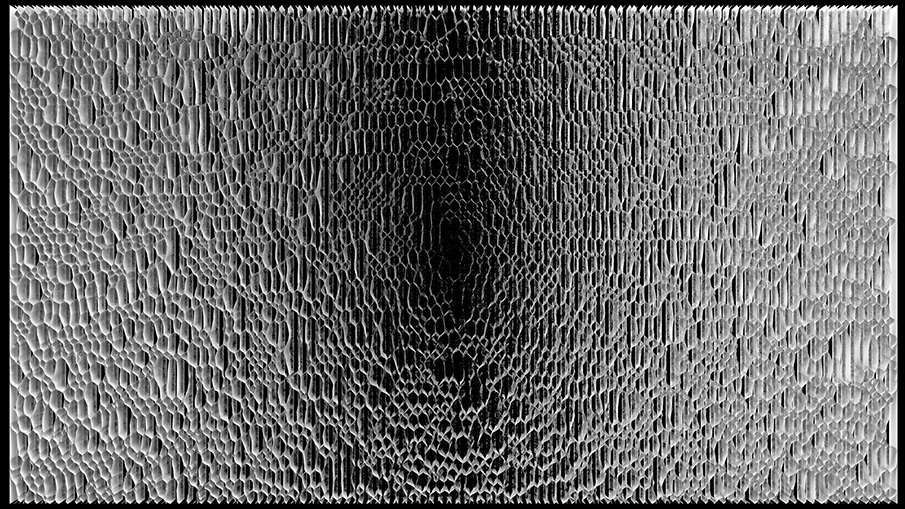

How to draw a line through the digital? How to draw a line not through a metaphorical reading of social, industrial and economical shifts, but through the tectonics, structure and expression of the digital itself? A formulation starts with the declaration of terms and a set of axioms.
When in geometry a line is described by a set of axioms, the notion of a line is usually left undefined (a so-called primitive object). The properties of lines are then determined by the axioms which refer to them. (...) In an axiomatic formulation of Euclidean geometry, such as that of Hilbert a line is stated to have certain properties which relate it to other lines and points.
Obviously, architecture is not geometry, but it extends it. It builds on geometry by a cartesian (a) colocation and (b) disposition of its parts. For architecture as composition its parts are defined as designed objects. Withdrawn, the designed object becomes congruent to the geometrical term of a point. The term of a point must than be congruent to the architectural view of a designed object. The term point has to be extended by (a) thickness as containment of that which is in. Additionally axioms have to describe (b) directionality as that in which it is. Then architecture as composition is nothing more than the difference between (a) being-inside and (b) being-outside.
For architecture (a) a term is the colocation of that which is in. (b) A axiom is that in which it is: the premise how to dispose.
specific architectural extensions:
For our design approach we declare as terms: Points, Segments and Lines.
(a) terms:
(1) a point has a thickness defined by a certain weight as a factor influencing dispositional operations.
(2) a segment has a thickness defined as a certain offset perpendicular to its length.
(3) a line is a certain composition of segments.
(b) axioms:
axioms of incidence:
(a) Every line contains minimum one line segment.
(b) Every segment contains exactly two points.
(c) For every point on a line except of two points exists exactly two segments which contain that point.
axioms of order:
(a) Every line is limited by its contained segments.
(b) Every segment is bounded by its contained points.
axioms of congruence:
(a) The segments contained by a line are not congruent to each other.
(b) The two points contained by a line segment are not congruent to each other.
(c) Points are immutable. Congruence means comparability; not the same reference.
axioms of composition:
(1) An Overlap of two segments, not contained by the same line, produce a new line, containing the points of the overlapping segments.